Portal:Mathematics

The Mathematics Portal

Isaac Newton

Mathematics is the study of representing and reasoning about abstract objects (such as numbers, points, spaces, sets, structures, and games). Mathematics is used throughout the world as an essential tool in many fields, including natural science, engineering, medicine, and the social sciences. Applied mathematics, the branch of mathematics concerned with application of mathematical knowledge to other fields, inspires and makes use of new mathematical discoveries and sometimes leads to the development of entirely new mathematical disciplines, such as statistics and game theory. Mathematicians also engage in pure mathematics, or mathematics for its own sake, without having any application in mind. There is no clear line separating pure and applied mathematics, and practical applications for what began as pure mathematics are often discovered. (Full article...)

Featured articles –

  Featured articles are displayed here, which represent some of the best content on English Wikipedia.

---

• 
  
  

  

  A stamp of Zhang Heng issued by China Post in 1955

  
  Zhang Heng (Chinese: 張衡; AD 78–139), formerly romanized as Chang Heng, was a Chinese polymathic scientist and statesman who lived during the Han dynasty. Educated in the capital cities of Luoyang and Chang'an, he achieved success as an astronomer, mathematician, seismologist, hydraulic engineer, inventor, geographer, cartographer, ethnographer, artist, poet, philosopher, politician, and literary scholar.
  
  Zhang Heng began his career as a minor civil servant in Nanyang. Eventually, he became Chief Astronomer, Prefect of the Majors for Official Carriages, and then Palace Attendant at the imperial court. His uncompromising stance on historical and calendrical issues led to his becoming a controversial figure, preventing him from rising to the status of Grand Historian. His political rivalry with the palace eunuchs during the reign of Emperor Shun (r. 125–144) led to his decision to retire from the central court to serve as an administrator of Hejian Kingdom in present-day Hebei. Zhang returned home to Nanyang for a short time, before being recalled to serve in the capital once more in 138. He died there a year later, in 139. (Full article...)
• 

  

  The manipulations of the Rubik's Cube form the Rubik's Cube group.

  
  In mathematics, a group is a set with an operation that satisfies the following constraints: the operation is associative and has an identity element, and every element of the set has an inverse element.
  
  Many mathematical structures are groups endowed with other properties. For example, the integers with the addition operation form an infinite group, which is generated by a single element called 1 (these properties characterize the integers in a unique way). (Full article...)
• 
  
  

  

  Archimedes Thoughtful
  by Domenico Fetti (1620)

  
  Archimedes of Syracuse (/ˌɑːrkɪˈmiːdiːz/, ARK-ihm-EE-deez; c. 287 – c. 212 BC) was an Ancient Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Considered the greatest mathematician of ancient history, and one of the greatest of all time, Archimedes anticipated modern calculus and analysis by applying the concept of the infinitely small and the method of exhaustion to derive and rigorously prove a range of geometrical theorems. These include the area of a circle, the surface area and volume of a sphere, the area of an ellipse, the area under a parabola, the volume of a segment of a paraboloid of revolution, the volume of a segment of a hyperboloid of revolution, and the area of a spiral.
  
  Archimedes' other mathematical achievements include deriving an approximation of pi, defining and investigating the Archimedean spiral, and devising a system using exponentiation for expressing very large numbers. He was also one of the first to apply mathematics to physical phenomena, working on statics and hydrostatics. Archimedes' achievements in this area include a proof of the law of the lever, the widespread use of the concept of center of gravity, and the enunciation of the law of buoyancy known as Archimedes' principle. He is also credited with designing innovative machines, such as his screw pump, compound pulleys, and defensive war machines to protect his native Syracuse from invasion. (Full article...)
• 
  In classical mechanics, the Laplace–Runge–Lenz (LRL) vector is a vector used chiefly to describe the shape and orientation of the orbit of one astronomical body around another, such as a binary star or a planet revolving around a star. For two bodies interacting by Newtonian gravity, the LRL vector is a constant of motion, meaning that it is the same no matter where it is calculated on the orbit; equivalently, the LRL vector is said to be conserved. More generally, the LRL vector is conserved in all problems in which two bodies interact by a central force that varies as the inverse square of the distance between them; such problems are called Kepler problems.
  
  The hydrogen atom is a Kepler problem, since it comprises two charged particles interacting by Coulomb's law of electrostatics, another inverse-square central force. The LRL vector was essential in the first quantum mechanical derivation of the spectrum of the hydrogen atom, before the development of the Schrödinger equation. However, this approach is rarely used today. (Full article...)
• 
  General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalises special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the curvature of spacetime is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second order partial differential equations.
  
  Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gravitation in classical physics. These predictions concern the passage of time, the geometry of space, the motion of bodies in free fall, and the propagation of light, and include gravitational time dilation, gravitational lensing, the gravitational redshift of light, the Shapiro time delay and singularities/black holes. So far, all tests of general relativity have been shown to be in agreement with the theory. The time-dependent solutions of general relativity enable us to talk about the history of the universe and have provided the modern framework for cosmology, thus leading to the discovery of the Big Bang and cosmic microwave background radiation. Despite the introduction of a number of alternative theories, general relativity continues to be the simplest theory consistent with experimental data. (Full article...)
• 

  

  Plots of logarithm functions, with three commonly used bases. The special points log_b b = 1 are indicated by dotted lines, and all curves intersect in log_b 1 = 0.

  
  
  In mathematics, the logarithm is the inverse function to exponentiation. That means that the logarithm of a number x to the base b is the exponent to which b must be raised to produce x. For example, since 1000 = 10³, the logarithm base 10 of 1000 is 3, or log₁₀ (1000) = 3. The logarithm of x to base b is denoted as log_b (x), or without parentheses, log_b x, or even without the explicit base, log x, when no confusion is possible, or when the base does not matter such as in big O notation.
  
  The logarithm base 10 is called the decimal or common logarithm and is commonly used in science and engineering. The natural logarithm has the number e ≈ 2.718 as its base; its use is widespread in mathematics and physics, because of its very simple derivative. The binary logarithm uses base 2 and is frequently used in computer science. (Full article...)
• 
  
  

  

  Damage from Hurricane Katrina in 2005. Actuaries need to estimate long-term levels of such damage in order to accurately price property insurance, set appropriate reserves, and design appropriate reinsurance and capital management strategies.

  
  An actuary is a professional with advanced mathematical skills who deals with the measurement and management of risk and uncertainty. The name of the corresponding field is actuarial science which covers rigorous mathematical calculations in areas of life expectancy and life insurance. These risks can affect both sides of the balance sheet and require asset management, liability management, and valuation skills. Actuaries provide assessments of financial security systems, with a focus on their complexity, their mathematics, and their mechanisms.
  
  While the concept of insurance dates to antiquity, the concepts needed to scientifically measure and mitigate risks have their origins in the 17th century studies of probability and annuities. Actuaries of the 21st century require analytical skills, business knowledge, and an understanding of human behavior and information systems to design and manage programs that control risk. The actual steps needed to become an actuary are usually country-specific; however, almost all processes share a rigorous schooling or examination structure and take many years to complete. (Full article...)
• 

  

  Logic studies valid forms of inference like modus ponens.

  
  Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the science of deductively valid inferences or logical truths. It studies how conclusions follow from premises due to the structure of arguments alone, independent of their topic and content. Informal logic is associated with informal fallacies, critical thinking, and argumentation theory. It examines arguments expressed in natural language while formal logic uses formal language. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Logic plays a central role in many fields, such as philosophy, mathematics, computer science, and linguistics.
  
  Logic studies arguments, which consist of a set of premises together with a conclusion. An example is the argument from the premises "it's Sunday" and "if it's Sunday then I don't have to work" to the conclusion "I don't have to work". Premises and conclusions express propositions or claims that can be true or false. An important feature of propositions is their internal structure. For example, complex propositions are made up of simpler propositions linked by logical vocabulary like $\land$ (and) or $\to$ (if...then). Simple propositions also have parts, like "Sunday" or "work" in the example. The truth of a proposition usually depends on the meanings of all of its parts. However, this is not the case for logically true propositions. They are true only because of their logical structure independent of the specific meanings of the individual parts. (Full article...)
• 
  
  

  

  Portrait by Jakob Emanuel Handmann, 1753

  
  Leonhard Euler (/ˈɔɪlər/ OY-lər, German: [ˈleːɔnhaʁt ˈʔɔʏlɐ] ^ⓘ, Swiss Standard German: [ˈleːɔnhart ˈɔʏlər]; 15 April 1707 – 18 September 1783) was a Swiss mathematician, physicist, astronomer, geographer, logician, and engineer who founded the studies of graph theory and topology and made pioneering and influential discoveries in many other branches of mathematics such as analytic number theory, complex analysis, and infinitesimal calculus. He introduced much of modern mathematical terminology and notation, including the notion of a mathematical function. He is also known for his work in mechanics, fluid dynamics, optics, astronomy, and music theory.
  
  Euler is held to be one of the greatest mathematicians in history and the greatest of the 18th century. Several great mathematicians who produced their work after Euler's death have recognised his importance in the field as shown by quotes attributed to many of them: Pierre-Simon Laplace expressed Euler's influence on mathematics by stating, "Read Euler, read Euler, he is the master of us all." Carl Friedrich Gauss wrote: "The study of Euler's works will remain the best school for the different fields of mathematics, and nothing else can replace it." Euler is also widely considered to be the most prolific; his 866 publications as well as his correspondences are being collected in the Opera Omnia Leonhard Euler which, when completed, will consist of 81 quarto volumes. He spent most of his adult life in Saint Petersburg, Russia, and in Berlin, then the capital of Prussia. (Full article...)
• 
  
  

  

  The title page of a 1634 version of Hues' Tractatus de globis in the collection of the Biblioteca Nacional de Portugal

  
  Robert Hues (1553 – 24 May 1632) was an English mathematician and geographer. He attended St. Mary Hall at Oxford, and graduated in 1578. Hues became interested in geography and mathematics, and studied navigation at a school set up by Walter Raleigh. During a trip to Newfoundland, he made observations which caused him to doubt the accepted published values for variations of the compass. Between 1586 and 1588, Hues travelled with Thomas Cavendish on a circumnavigation of the globe, performing astronomical observations and taking the latitudes of places they visited. Beginning in August 1591, Hues and Cavendish again set out on another circumnavigation of the globe. During the voyage, Hues made astronomical observations in the South Atlantic, and continued his observations of the variation of the compass at various latitudes and at the Equator. Cavendish died on the journey in 1592, and Hues returned to England the following year.
  
  In 1594, Hues published his discoveries in the Latin work Tractatus de globis et eorum usu (Treatise on Globes and Their Use) which was written to explain the use of the terrestrial and celestial globes that had been made and published by Emery Molyneux in late 1592 or early 1593, and to encourage English sailors to use practical astronomical navigation. Hues' work subsequently went into at least 12 other printings in Dutch, English, French and Latin. (Full article...)
• 
  The number π (/paɪ/; spelled out as "pi") is a mathematical constant that is the ratio of a circle's circumference to its diameter, approximately equal to 3.14159. The number π appears in many formulae across mathematics and physics. It is an irrational number, meaning that it cannot be expressed exactly as a ratio of two integers, although fractions such as ${\tfrac {22}{7}}$ are commonly used to approximate it. Consequently, its decimal representation never ends, nor enters a permanently repeating pattern. It is a transcendental number, meaning that it cannot be a solution of an equation involving only finite sums, products, powers, and integers. The transcendence of π implies that it is impossible to solve the ancient challenge of squaring the circle with a compass and straightedge. The decimal digits of π appear to be randomly distributed, but no proof of this conjecture has been found.
  
  For thousands of years, mathematicians have attempted to extend their understanding of π, sometimes by computing its value to a high degree of accuracy. Ancient civilizations, including the Egyptians and Babylonians, required fairly accurate approximations of π for practical computations. Around 250 BC, the Greek mathematician Archimedes created an algorithm to approximate π with arbitrary accuracy. In the 5th century AD, Chinese mathematicians approximated π to seven digits, while Indian mathematicians made a five-digit approximation, both using geometrical techniques. The first computational formula for π, based on infinite series, was discovered a millennium later. The earliest known use of the Greek letter π to represent the ratio of a circle's circumference to its diameter was by the Welsh mathematician William Jones in 1706. (Full article...)
• 
  
  

  

  Rejewski, c. 1932

  
  Marian Adam Rejewski (Polish: [ˈmarjan rɛˈjɛfskʲi] ^ⓘ; 16 August 1905 – 13 February 1980) was a Polish mathematician and cryptologist who in late 1932 reconstructed the sight-unseen Nazi German military Enigma cipher machine, aided by limited documents obtained by French military intelligence. Over the next nearly seven years, Rejewski and fellow mathematician-cryptologists Jerzy Różycki and Henryk Zygalski developed and used techniques and equipment to decrypt the German machine ciphers, even as the Germans introduced modifications to their equipment and encryption procedures.
  
  Five weeks before the outbreak of World War II in Europe, the Poles shared their technological achievements with the French and British at a conference in Warsaw, thus enabling Britain to begin reading German Enigma-encrypted messages, seven years after Rejewski's original reconstruction of the machine. The intelligence that was gained by the British from Enigma decrypts formed part of what was code-named Ultra and contributed—perhaps decisively—to the defeat of Nazi Germany. (Full article...)
• 
  
  

  

  Title page of the first edition of Wright's Certaine Errors in Navigation (1599)

  
  Edward Wright (baptised 8 October 1561; died November 1615) was an English mathematician and cartographer noted for his book Certaine Errors in Navigation (1599; 2nd ed., 1610), which for the first time explained the mathematical basis of the Mercator projection by building on the works of Pedro Nunes, and set out a reference table giving the linear scale multiplication factor as a function of latitude, calculated for each minute of arc up to a latitude of 75°. This was in fact a table of values of the integral of the secant function, and was the essential step needed to make practical both the making and the navigational use of Mercator charts.
  
  Wright was born at Garveston in Norfolk and educated at Gonville and Caius College, Cambridge, where he became a fellow from 1587 to 1596. In 1589 the college granted him leave after Elizabeth I requested that he carry out navigational studies with a raiding expedition organised by the Earl of Cumberland to the Azores to capture Spanish galleons. The expedition's route was the subject of the first map to be prepared according to Wright's projection, which was published in Certaine Errors in 1599. The same year, Wright created and published the first world map produced in England and the first to use the Mercator projection since Gerardus Mercator's original 1569 map. (Full article...)
• 

  

  High-precision test of general relativity by the Cassini space probe (artist's impression): radio signals sent between the Earth and the probe (green wave) are delayed by the warping of spacetime (blue lines) due to the Sun's mass.

  
  
  General relativity is a theory of gravitation developed by Albert Einstein between 1907 and 1915. The theory of general relativity says that the observed gravitational effect between masses results from their warping of spacetime.
  
  By the beginning of the 20th century, Newton's law of universal gravitation had been accepted for more than two hundred years as a valid description of the gravitational force between masses. In Newton's model, gravity is the result of an attractive force between massive objects. Although even Newton was troubled by the unknown nature of that force, the basic framework was extremely successful at describing motion. (Full article...)
• 

  

  The first 15,000 partial sums of 0 + 1 − 2 + 3 − 4 + ... The graph is situated with positive integers to the right and negative integers to the left.

  
  
  In mathematics, 1 − 2 + 3 − 4 + ··· is an infinite series whose terms are the successive positive integers, given alternating signs. Using sigma summation notation the sum of the first m terms of the series can be expressed as
  

  $$\sum _{n=1}^{m}n(-1)^{n-1}.$$

  
  
  The infinite series diverges, meaning that its sequence of partial sums, (1, −1, 2, −2, 3, ...), does not tend towards any finite limit. Nonetheless, in the mid-18th century, Leonhard Euler wrote what he admitted to be a paradoxical equation:
  

  $$1-2+3-4+\cdots ={\frac {1}{4}}.$$

  (Full article...)

Selected image –

graph showing two sets of 4 points, each set perfectly fit by a trend line with positive slope; the set of points on the left is higher and the set on the right lower, so the entire collection of points is best fit by a trend line with negative slope

Simpson's paradox

Credit: Schutz

Simpson's paradox (also known as the Yule–Simpson effect) states that an observed association between two variables can reverse when considered at separate levels of a third variable (or, conversely, that the association can reverse when separate groups are combined). Shown here is an illustration of the paradox for quantitative data. In the graph the overall association between X and Y is negative (as X increases, Y tends to decrease when all of the data is considered, as indicated by the negative slope of the dashed line); but when the blue and red points are considered separately (two levels of a third variable, color), the association between X and Y appears to be positive in each subgroup (positive slopes on the blue and red lines — note that the effect in real-world data is rarely this extreme). Named after British statistician Edward H. Simpson, who first described the paradox in 1951 (in the context of qualitative data), similar effects had been mentioned by Karl Pearson (and coauthors) in 1899, and by Udny Yule in 1903. One famous real-life instance of Simpson's paradox occurred in the UC Berkeley gender-bias case of the 1970s, in which the university was sued for gender discrimination because it had a higher admission rate for male applicants to its graduate schools than for female applicants (and the effect was statistically significant). The effect was reversed, however, when the data was split by department: most departments showed a small but significant bias in favor of women. The explanation was that women tended to apply to competitive departments with low rates of admission even among qualified applicants, whereas men tended to apply to less-competitive departments with high rates of admission among qualified applicants. (Note that splitting by department was a more appropriate way of looking at the data since it is individual departments, not the university as a whole, that admit graduate students.)

Good articles –

  These are Good articles, which meet a core set of high editorial standards.

---

• 

  

  The complete graph K₄ has ten matchings, corresponding to the value T(4) = 10 of the fourth telephone number.

  
  In mathematics, the telephone numbers or the involution numbers form a sequence of integers that count the ways n people can be connected by person-to-person telephone calls. These numbers also describe the number of matchings (the Hosoya index) of a complete graph on n vertices, the number of permutations on n elements that are involutions, the sum of absolute values of coefficients of the Hermite polynomials, the number of standard Young tableaux with n cells, and the sum of the degrees of the irreducible representations of the symmetric group. Involution numbers were first studied in 1800 by Heinrich August Rothe, who gave a recurrence equation by which they may be calculated, giving the values (starting from n = 0) (Full article...)
• 
  
  

  

  

  Two symmetric orthographic projections

  
  In geometry, the complete or final stellation of the icosahedron is the outermost stellation of the icosahedron, and is "complete" and "final" because it includes all of the cells in the icosahedron's stellation diagram. That is, every three intersecting face planes of the icosahedral core intersect either on a vertex of this polyhedron or inside of it. It was studied by Max Brückner after the discovery of Kepler–Poinsot polyhedron. It can be viewed as an irregular, simple, and star polyhedron. (Full article...)
• 

  

  Two intersecting families of two-element subsets of a four-element set. The sets in the left family all contain the bottom left element. The sets in the right family avoid this element.

  
  In mathematics, the Erdős–Ko–Rado theorem limits the number of sets in a family of sets for which every two sets have at least one element in common. Paul Erdős, Chao Ko, and Richard Rado proved the theorem in 1938, but did not publish it until 1961. It is part of the field of combinatorics, and one of the central results of extremal set theory.
  The theorem applies to families of sets that all have the same size, $r$, and are all subsets of some larger set of size $n$. One way to construct a family of sets with these parameters, each two sharing an element, is to choose a single element to belong to all the subsets, and then form all of the subsets that contain the chosen element. The Erdős–Ko–Rado theorem states that when $n$ is large enough for the problem to be nontrivial ($n\geq 2r$) this construction produces the largest possible intersecting families. When $n=2r$ there are other equally-large families, but for larger values of $n$ only the families constructed in this way can be largest. (Full article...)
• 

  

  
  Jessen's icosahedron, sometimes called Jessen's orthogonal icosahedron, is a non-convex polyhedron with the same numbers of vertices, edges, and faces as the regular icosahedron. It is named for Børge Jessen, who studied it in 1967. In 1971, a family of nonconvex polyhedra including this shape was independently discovered and studied by Adrien Douady under the name six-beaked shaddock; later authors have applied variants of this name more specifically to Jessen's icosahedron.
  
  The faces of Jessen's icosahedron meet only in right angles, even though it has no orientation where they are all parallel to the coordinate planes. It is a "shaky polyhedron", meaning that (like a flexible polyhedron) it is not infinitesimally rigid. Outlining the edges of this polyhedron with struts and cables produces a widely-used tensegrity structure, also called the six-bar tensegrity, tensegrity icosahedron, or expanded octahedron. (Full article...)
• 

  

  A set of 20 points in a 10 × 10 grid, with no three points in a line.

  
  The no-three-in-line problem in discrete geometry asks how many points can be placed in the $n\times n$ grid so that no three points lie on the same line. The problem concerns lines of all slopes, not only those aligned with the grid. It was introduced by Henry Dudeney in 1900. Brass, Moser, and Pach call it "one of the oldest and most extensively studied geometric questions concerning lattice points".
  
  At most $2n$ points can be placed, because $2n+1$ points in a grid would include a row of three or more points, by the pigeonhole principle. Although the problem can be solved with $2n$ points for every $n$ up to $46$, it is conjectured that fewer than $2n$ points can be placed in grids of large size. Known methods can place linearly many points in grids of arbitrary size, but the best of these methods place slightly fewer than $3n/2$ points, not $2n$. (Full article...)
• 
  The Alexandrov uniqueness theorem is a rigidity theorem in mathematics, describing three-dimensional convex polyhedra in terms of the distances between points on their surfaces. It implies that convex polyhedra with distinct shapes from each other also have distinct metric spaces of surface distances, and it characterizes the metric spaces that come from the surface distances on polyhedra. It is named after Soviet mathematician Aleksandr Danilovich Aleksandrov, who published it in the 1940s. (Full article...)
• 
  Donkey Kong Jr. Math is an edutainment platform video game developed and published by Nintendo for the Nintendo Entertainment System. It is a spin-off of the 1982 arcade game Donkey Kong Jr. In the game, players control Donkey Kong Jr. as he solves math problems set up by his father Donkey Kong. It was released in Japan in 1983 for the Family Computer, and in North America and the PAL region in 1986.
  
  It is the only game in the Education Series of NES games in North America, owing to the game's lack of success. It was made available in various forms, including in the 2002 GameCube video game Animal Crossing and on the Virtual Console services for Wii and Wii U in 2007 and 2014 respectively. Donkey Kong Jr. Math was a critical and commercial failure. It has received criticism from several publications including IGN staff, who called it one of the worst Virtual Console games. (Full article...)
• 

  

  The maximum spacing method tries to find a distribution function such that the spacings, D_(i), are all approximately of the same length. This is done by maximizing their geometric mean.

  
  
  In statistics, maximum spacing estimation (MSE or MSP), or maximum product of spacing estimation (MPS), is a method for estimating the parameters of a univariate statistical model. The method requires maximization of the geometric mean of spacings in the data, which are the differences between the values of the cumulative distribution function at neighbouring data points.
  
  The concept underlying the method is based on the probability integral transform, in that a set of independent random samples derived from any random variable should on average be uniformly distributed with respect to the cumulative distribution function of the random variable. The MPS method chooses the parameter values that make the observed data as uniform as possible, according to a specific quantitative measure of uniformity. (Full article...)
• 

  

  The Shapley–Folkman lemma is illustrated by the Minkowski addition of four sets. The point (+) in the convex hull of the Minkowski sum of the four non-convex sets (right) is the sum of four points (+) from the (left-hand) sets—two points in two non-convex sets plus two points in the convex hulls of two sets. The convex hulls are shaded pink. The original sets each have exactly two points (shown as red dots).

  
  The Shapley–Folkman lemma is a result in convex geometry that describes the Minkowski addition of sets in a vector space. It is named after mathematicians Lloyd Shapley and Jon Folkman, but was first published by the economist Ross M. Starr.
  
  The lemma may be intuitively understood as saying that, if the number of summed sets exceeds the dimension of the vector space, then their Minkowski sum is approximately convex. (Full article...)
• 

  

  A one-dimensional reversible cellular automaton with nine states. At each step, each cell copies the shape from its left neighbor, and the color from its right neighbor.

  
  A reversible cellular automaton is a cellular automaton in which every configuration has a unique predecessor. That is, it is a regular grid of cells, each containing a state drawn from a finite set of states, with a rule for updating all cells simultaneously based on the states of their neighbors, such that the previous state of any cell before an update can be determined uniquely from the updated states of all the cells. The time-reversed dynamics of a reversible cellular automaton can always be described by another cellular automaton rule, possibly on a much larger neighborhood.
  
  Several methods are known for defining cellular automata rules that are reversible; these include the block cellular automaton method, in which each update partitions the cells into blocks and applies an invertible function separately to each block, and the second-order cellular automaton method, in which the update rule combines states from two previous steps of the automaton. When an automaton is not defined by one of these methods, but is instead given as a rule table, the problem of testing whether it is reversible is solvable for block cellular automata and for one-dimensional cellular automata, but is undecidable for other types of cellular automata. (Full article...)
• 
  In mathematics, the harmonic series is the infinite series formed by summing all positive unit fractions:
  

  $$\sum _{n=1}^{\infty }{\frac {1}{n}}=1+{\frac {1}{2}}+{\frac {1}{3}}+{\frac {1}{4}}+{\frac {1}{5}}+\cdots .$$

  
  
  The first $n$ terms of the series sum to approximately $\ln n+\gamma$, where $\ln$ is the natural logarithm and $\gamma \approx 0.577$ is the Euler–Mascheroni constant. Because the logarithm has arbitrarily large values, the harmonic series does not have a finite limit: it is a divergent series. Its divergence was proven in the 14th century by Nicole Oresme using a precursor to the Cauchy condensation test for the convergence of infinite series. It can also be proven to diverge by comparing the sum to an integral, according to the integral test for convergence. (Full article...)
• 

  

  In this example, the alternating sum of angles (clockwise from the bottom) is 90° − 45° + 22.5° − 22.5° + 45° − 90° + 22.5° − 22.5° = 0°. Since it adds to zero, the crease pattern may be flat-folded.

  
  Kawasaki's theorem or Kawasaki–Justin theorem is a theorem in the mathematics of paper folding that describes the crease patterns with a single vertex that may be folded to form a flat figure. It states that the pattern is flat-foldable if and only if alternatingly adding and subtracting the angles of consecutive folds around the vertex gives an alternating sum of zero.
  Crease patterns with more than one vertex do not obey such a simple criterion, and are NP-hard to fold.
  
  The theorem is named after one of its discoverers, Toshikazu Kawasaki. However, several others also contributed to its discovery, and it is sometimes called the Kawasaki–Justin theorem or Husimi's theorem after other contributors, Jacques Justin and Kôdi Husimi. (Full article...)

Did you know (auto-generated) –

• ... that some philosophers of mathematics believe that the life cycle of a species of cicadas is a good argument for the existence of numbers?
• ... that The Math Myth advocates for American high schools to stop requiring advanced algebra?
• ... that the 1914 Lubin vault fire in Philadelphia destroyed several thousand unique early silent films?
• ... that in the aftermath of the American Civil War, the only Black-led organization providing teachers to formerly enslaved people was the African Civilization Society?
• ... that multiple mathematics competitions have made use of Sophie Germain's identity?
• ... that the number of cannonballs in a square pyramid with $n$ cannonballs along each edge is ${\frac {n(n+1)(2n+1)}{6}}$?
• ... that museum director Alena Aladava rebuilt the Belarusian national art collection in the aftermath of the Second World War?
• ... that the mathematical infinity symbol ∞ may be derived from the Roman numerals for 1000 or for 100 million?

More did you know –

Did you know...

• ...that the mathematician Grigori Perelman was offered a Fields Medal in 2006, in part for his proof of the Poincaré conjecture, which he declined?
• ...that a regular heptagon is the regular polygon with the fewest sides which is not constructible with a compass and straightedge?
• ...that the regular trigonometric functions and the hyperbolic trigonometric functions can be related without using complex numbers through the Gudermannian function?
• ...that the Catalan numbers solve a number of problems in combinatorics such as the number of ways to completely parenthesize an algebraic expression with n+1 factors?
• ...that a ball can be cut up and reassembled into two balls, each the same size as the original (Banach-Tarski paradox)?
• ...that it is impossible to devise a single formula involving only polynomials and radicals for solving an arbitrary quintic equation?
• ...that Euler found 59 more amicable numbers while for 2000 years, only 3 pairs had been found before him?

Selected article –

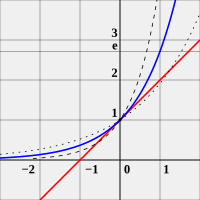e is the unique number such that the slope of y=ex (blue curve) is exactly 1 when x=0 (illustrated by the red tangent line). For comparison, the curves y=2x (dotted curve) and y=4x (dashed curve) are shown. Image credit: Dick Lyon

The mathematical constant e is occasionally called Euler's number after the Swiss mathematician Leonhard Euler, or Napier's constant in honor of the Scottish mathematician John Napier who introduced logarithms. It is one of the most important numbers in mathematics, alongside the additive and multiplicative identities 0 and 1, the imaginary unit i, and π, the circumference to diameter ratio for any circle. It has a number of equivalent definitions. One is given in the caption of the image to the right, and three more are:

1. The sum of the infinite series

   ${\begin{aligned}e&=\sum _{n=0}^{\infty }{\frac {1}{n!}}\\&={\frac {1}{0!}}+{\frac {1}{1!}}+{\frac {1}{2!}}+{\frac {1}{3!}}+\cdots \\\end{aligned}}$

   where n! is the factorial of n, and 0! is defined to be 1 by convention.

2. The global maximizer of the function

   $f(x)=x^{1 \over x}.$

3. The limit:

   $e=\lim _{n\to \infty }\left(1+{\frac {1}{n}}\right)^{n}$

The number e is also the base of the natural logarithm. Since e is transcendental, and therefore irrational, its value can not be given exactly. The numerical value of e truncated to 20 decimal places is 2.71828 18284 59045 23536. (Full article...)

View all selected articles

Subcategories

Algebra | Arithmetic | Analysis | Complex analysis | Applied mathematics | Calculus | Category theory | Chaos theory | Combinatorics | Dynamical systems | Fractals | Game theory | Geometry | Algebraic geometry | Graph theory | Group theory | Linear algebra | Mathematical logic | Model theory | Multi-dimensional geometry | Number theory | Numerical analysis | Optimization | Order theory | Probability and statistics | Set theory | Statistics | Topology | Algebraic topology | Trigonometry | Linear programming

---

Mathematics | History of mathematics | Mathematicians | Awards | Education | Literature | Notation | Organizations | Theorems | Proofs | Unsolved problems

Full category tree. Select [►] to view subcategories.

Mathematics

Mathematics by country

Fields of mathematics

Mathematics-related lists

Mathematicians

Works about mathematics

Mathematics and art

Mathematical classification systems

Mathematical concepts

Mathematical constants

Mathematics and culture

Eponyms in mathematics

Mathematical examples

History of mathematics

Mathematical notation

Mathematical science occupations

Outlines of mathematics and logic

Philosophy of mathematics

Mathematical projects

Mathematical proofs

Pseudomathematics

Set index articles on mathematics

Mathematical terminology

Mathematical theorems

Mathematical tools

Mathematics stubs

Topics in mathematics

General	Foundations	Number theory	Discrete mathematics
Mathematicians History of mathematics Philosophy of mathematics Mathematical notation Mathematical beauty Mathematics education Areas of mathematics Outline of mathematics Glossary of mathematical symbols	Foundations of mathematics Mathematical logic Proof theory Gödel's incompleteness theorems Model theory Recursion theory Set theory Naive set theory Axiomatic set theory Category theory Topos theory	Number theory Algebraic number theory Analytic number theory Arithmetic Fundamental theorem of arithmetic Numbers Natural numbers Prime numbers Rational numbers Algebraic numbers	Discrete mathematics Combinatorics Combinatorial geometry Coding theory Combinatorial design Enumerative combinatorics Combinatorial optimization Graph theory Order theory Lattice theory Digital Signal Processing Theoretical computer science Computability theory Complexity theory
Algebra	Analysis	Geometry and topology	Applied mathematics
Algebra Elementary algebra Abstract algebra Group theory Ring theory Field theory Commutative algebra Geometric algebra Linear algebra Matrix theory Multilinear algebra Universal algebra Fundamental theorem of algebra	Analysis Calculus Fundamental theorem of calculus Vector calculus Geometric calculus Measure theory Real analysis Complex analysis Differential equations Ordinary differential equations Partial differential equations Integral equations Approximation theory Special functions Potential theory Harmonic analysis Fourier analysis Functional analysis Operator theory	Geometry Euclidean geometry Trigonometry Analytic geometry Non-Euclidean geometry Affine geometry Projective geometry Convex geometry Discrete geometry Algebraic geometry Differential geometry K theory Gauge theory Riemannian geometry Lie groups Topology General topology Algebraic topology Geometric topology Differential topology	Applied mathematics Mathematical modeling Mathematical physics Dynamical systems Control theory Calculus of variations Optimization Mathematical economics Game theory Mathematical finance Statistics Probability theory Stochastic processes Numerical analysis Cryptography Information theory

Index of mathematics articles

ARTICLE INDEX:	0–9 A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
MATHEMATICIANS:	A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

Related portals

WikiProjects

The Mathematics WikiProject is the center for mathematics-related editing on Wikipedia. Join the discussion on the project's talk page.

Project pages

• Participants
• Current activity
• Manual of style
• Conventions

Essays

• Advice on using Wikipedia for mathematics self-study
• Proofs

Subprojects

• PlanetMath Exchange (Talk)
• Graphics (Talk)
• Article assessment (Talk)

Related projects

Computer science | Cryptography | Game theory | Numbers | Physics | Science | Statistics

Things you can do

• Create a new article: List of requested articles
• Add a new image: Image requests
• Expand an article: Mathematics stubs
• Lend your expertise: Expert attention needed

In other Wikimedia projects

The following Wikimedia Foundation sister projects provide more on this subject:

• 

  Commons
  Free media repository

• 

  Wikibooks
  Free textbooks and manuals

• 

  Wikidata
  Free knowledge base

• 

  Wikinews
  Free-content news

• 

  Wikiquote
  Collection of quotations

• 

  Wikisource
  Free-content library

• 

  Wikiversity
  Free learning tools

• 

  Wiktionary
  Dictionary and thesaurus

More portals

Discover Wikipedia using portals

• 

  List of all portals

• 

  The arts portal

• 

  Biography portal

• 

  Current events portal

• 

  Geography portal

• 

  History portal

• 

  Mathematics portal

• 

  Science portal

• 

  Society portal

• 

  Technology portal

• 

  Random portal

• 

  WikiProject Portals